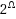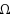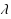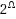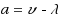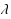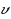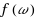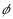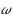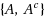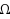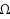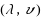In ordinary discourse the term ambiguity typically refers to vagueness or imprecision in a natural language. Among decision theorists, however, this term usually refers to imprecision in an individual’s probabilistic judgments, in the sense that the available evidence is consistent with more than one probability distribution over possible states of the world. Avoiding a prior commitment to either of these interpretations, Fishburn has explored ambiguity as a primitive concept, in terms of what he calls an ambiguity measure a on the power set
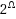 of a finite set
of a finite set
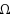 , characterized by five axioms. We prove, in purely set‐theoretic terms, that if
, characterized by five axioms. We prove, in purely set‐theoretic terms, that if
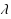 is a so‐called necessity measure on
is a so‐called necessity measure on
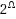 and
and
 is its associated possibility measure, then
is its associated possibility measure, then
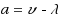 is an ambiguity measure. When
is an ambiguity measure. When
 is construed as a set of possible exemplars of a vague predicate
is construed as a set of possible exemplars of a vague predicate
 , then
, then
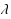 and
and
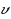 may be regarded as arising from a fuzzy membership function f on
may be regarded as arising from a fuzzy membership function f on
 , where
, where
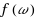 designates the degree to which
designates the degree to which
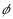 is applicable to
is applicable to
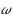 . In this case a(A) represents the degree to which the partition
. In this case a(A) represents the degree to which the partition
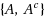 differentiates members of
differentiates members of
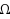 with respect to the predicate
with respect to the predicate
 . When
. When
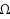 is construed as a set of possible states of the world, a necessity measure may be regarded as a very special type of lower probability known as a consonant belief function, and a possibility measure as its associated upper probability, whence a(A) represents the degree of imprecision in the pair
is construed as a set of possible states of the world, a necessity measure may be regarded as a very special type of lower probability known as a consonant belief function, and a possibility measure as its associated upper probability, whence a(A) represents the degree of imprecision in the pair
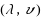 with respect to the event A. Fishburn’s axioms are thus consistent with an interpretation of ambiguity as linguistic vagueness, as well as (a very special sort of) probabilistic imprecision.
with respect to the event A. Fishburn’s axioms are thus consistent with an interpretation of ambiguity as linguistic vagueness, as well as (a very special sort of) probabilistic imprecision.