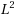A nonlinear weighted least‐squares finite element method for Stokes equations

The paper concerns a nonlinear weighted least‐squares finite element method for the solutions of the incompressible Stokes equations based on the application of the least‐squares minimization principle to an equivalent first order velocity–pressure–stress system. Model problem considered is the flow in a planar channel. The least‐squares functional involves the $L^2$ ‐norms of the residuals of each equation multiplied by a nonlinear weighting function and mesh dependent weights. Using linear approximations for all variables, by properly adjusting the importance of the mass conservation equation and a carefully chosen nonlinear weighting function, the least‐squares solutions exhibit optimal $L^2$ ‐norm error convergence in all unknowns. Numerical solutions of the flow pass through a 4 to 1 contraction channel will also be considered.