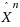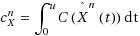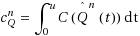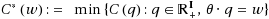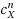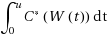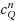A parallel server system is considered, with I customer classes and many servers, operating in a heavy traffic diffusion regime where the queueing delay and service time are of the same order of magnitude. Denoting by
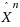 and
and
 , respectively, the diffusion scale deviation of the headcount process from the quantity corresponding to the underlying fluid model and the diffusion scale queue‐length, we consider minimizing r.v.’s of the form
, respectively, the diffusion scale deviation of the headcount process from the quantity corresponding to the underlying fluid model and the diffusion scale queue‐length, we consider minimizing r.v.’s of the form
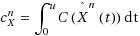 and
and
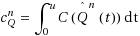 over policies that allow for service interruption. Here, C:ℝ
I→ℝ+ is continuous, and u>0. Denoting by θ the so‐called workload vector, it is assumed that
over policies that allow for service interruption. Here, C:ℝ
I→ℝ+ is continuous, and u>0. Denoting by θ the so‐called workload vector, it is assumed that
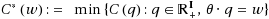 is attained along a continuous curve as w varies in ℝ+. We show that any weak limit point of
is attained along a continuous curve as w varies in ℝ+. We show that any weak limit point of
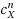 stochastically dominates the r.v.
stochastically dominates the r.v.
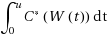 for a suitable reflected Brownian motion W and construct a sequence of policies that asymptotically achieve this lower bound. For
for a suitable reflected Brownian motion W and construct a sequence of policies that asymptotically achieve this lower bound. For
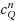 , an analogous result is proved when, in addition, C
* is convex. The construction of the policies takes full advantage of the fact that in this regime the number of servers is of the same order as the typical queue‐length.
, an analogous result is proved when, in addition, C
* is convex. The construction of the policies takes full advantage of the fact that in this regime the number of servers is of the same order as the typical queue‐length.