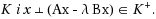| Article ID: | iaor20115113 |
| Volume: | 49 |
| Issue: | 2 |
| Start Page Number: | 299 |
| End Page Number: | 318 |
| Publication Date: | Jun 2011 |
| Journal: | Computational Optimization and Applications |
| Authors: | Seeger Alberto, Adly Samir |
| Keywords: | matrices |
We study several variants of a nonsmooth Newton‐type algorithm for solving an eigenvalue problem of the form