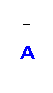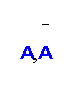How to deal with partially analyzable acts?

In some situations, a decision is best represented by an incompletely analyzed act: conditionally on a given event A, the consequences of the decision on sub‐events are perfectly known and uncertainty becomes probabilizable, whereas the plausibility of this event itself remains vague and the decision outcome on the complementary event 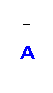$\overline{A}$ is imprecisely known. In this framework, we study an axiomatic decision model and prove a representation theorem. Resulting decision criteria aggregate partial evaluations consisting of (i) the conditional expected utility associated with the analyzed part of the decision, and (ii) the best and worst consequences of its non‐analyzed part. The representation theorem is consistent with a wide variety of decision criteria, which allows for expressing various degrees of knowledge on ( 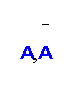$A,\overline{A}$ ) and various types of attitude toward ambiguity and uncertainty. This diversity is taken into account by specific models already existing in the literature. We exploit this fact and propose some particular forms of our model incorporating these models as sub‐models and moreover expressing various types of beliefs concerning the relative plausibility of the analyzed and the non‐analyzed events ranging from probabilities to complete ignorance that include capacities.