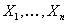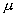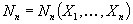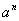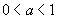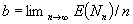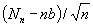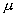| Article ID: | iaor19941809 |
| Country: | Netherlands |
| Volume: | 58 |
| Issue: | 2 |
| Start Page Number: | 229 |
| End Page Number: | 242 |
| Publication Date: | Feb 1993 |
| Journal: | Mathematical Programming (Series A) |
| Authors: | Talagrand Michel, Rhee WanSoo T. |
| Keywords: | probability |
Given a collection of items and a number of unit size bins, the dual bin packing problem requires finding the largest number of items that can be packed in these bins. In the present stochastic model, the item sizes