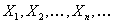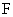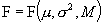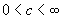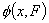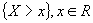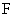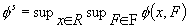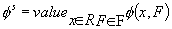| Article ID: | iaor19932349 |
| Country: | Japan |
| Volume: | 35 |
| Issue: | 1 |
| Start Page Number: | 1 |
| End Page Number: | 14 |
| Publication Date: | Mar 1992 |
| Journal: | Journal of the Operations Research Society of Japan |
| Authors: | Nakagami Jun-ichi |
| Keywords: | optimization, statistics: decision |
Let