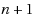In this paper, we consider an optimization problem with geometric and cone constraints, whose objective function is a composition of
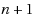functions. For this problem, we calculate its conjugate dual problem, where the functions involved in the objective function of the primal problem will be decomposed. Furthermore, we formulate generalized interior point regularity conditions for strong duality and give necessary and sufficient optimality conditions. As applications of this approach, we determine the formulas of the conjugate as well as the biconjugate of the objective function of the primal problem and discuss an optimization problem having as objective function the sum of reciprocals of concave functions.
