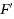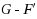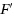| Article ID: | iaor20172777 |
| Volume: | 34 |
| Issue: | 2 |
| Start Page Number: | 522 |
| End Page Number: | 533 |
| Publication Date: | Aug 2017 |
| Journal: | Journal of Combinatorial Optimization |
| Authors: | Liu Yan, Liu Weiwei |
| Keywords: | combinatorial optimization, graphs, heuristics |
Let