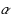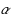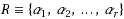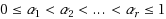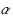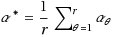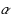| Article ID: | iaor20171809 |
| Volume: | 17 |
| Issue: | 1 |
| Start Page Number: | 239 |
| End Page Number: | 273 |
| Publication Date: | Apr 2017 |
| Journal: | Operational Research |
| Authors: | Xu Xiaojing, Ma Jinpeng, Xie Xiaoping |
| Keywords: | economics, investment, simulation, markov processes |
This paper studies a financial market transaction model and convergence of Markovian price processes generated by an