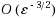Cubic-regularization counterpart of a variable-norm trust-region method for unconstrained minimization

In a recent paper, we introduced a trust‐region method with variable norms for unconstrained minimization, we proved standard asymptotic convergence results, and we discussed the impact of this method in global optimization. Here we will show that, with a simple modification with respect to the sufficient descent condition and replacing the trust‐region approach with a suitable cubic regularization, the complexity of this method for finding approximate first‐order stationary points is $O({\mathit{ϵ}}^{‐3/2})$ . We also prove a complexity result with respect to second‐order stationarity. Some numerical experiments are also presented to illustrate the effect of the modification on practical performance.