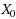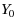| Article ID: | iaor20171753 |
| Volume: | 68 |
| Issue: | 2 |
| Start Page Number: | 329 |
| End Page Number: | 355 |
| Publication Date: | Jun 2017 |
| Journal: | Journal of Global Optimization |
| Authors: | Elser Veit |
| Keywords: | matrices, heuristics, numerical analysis |
The decomposition of a matrix, as a product of factors with particular properties, is a much used tool in numerical analysis. Here we develop methods for decomposing a matrix