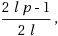An explicit analytic approximation of solutions for a class of neutral stochastic differential equations with time-dependent delay based on Taylor expansion

This paper represents a contribution to the analysis of approximate methods for stochastic differential equations based on the application of Taylor expansion, under the Lipschitz and linear growth conditions. The L^p and almost sure convergence of the appropriate approximate solutions are considered for a class of neutral stochastic differential equations with time‐dependent delay. Coefficients of the approximate equations, including the neutral term, are Taylor approximations of the coefficients of the initial equation up to the first derivatives. For p = 2, the rate of the L^p ‐convergence of the sequence of approximate solutions to the exact solution is estimated as $\frac{2lp‐1}{2l},$ where l > 1 is an integer. The presence of the neutral term in the equation reflected to the rate of convergence.