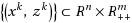Logarithmic quasi-distance proximal point scalarization method for multi-objective programming

Recently, Gregório and Oliveira developed a proximal point scalarization method (applied to multi‐objective optimization problems) for an abstract strict scalar representation with a variant of the logarithmic‐quadratic function of Auslender et al. as regularization. In this study, a variation of this method is proposed, using the regularization with logarithm and quasi‐distance. By restricting it to a certain class of quasi‐distances that are Lipschitz continuous and coercive in any of their arguments, we show that any sequence $\left\{(x^k,z^k)\right\}\subset R^n\times R_{++}^m$ generated by the method satisfies: {z^k } is convergent; and {x^k } is bounded and its accumulation points are weak Pareto solutions of the unconstrained multi‐objective optimization problem