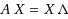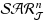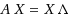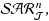The inverse eigenvalue problem of structured matrices from the design of Hopfield neural networks

By means of the properties of structured matrices from the design of Hopfield neural networks, we establish the necessary and sufficient conditions for the solvability of the inverse eigenvalue problem $AX=X\Lambda$ in structured matrix set ${\mathcal{SAR}}_{\mathcal{J}}^n$ . In the case where $AX=X\Lambda$ is solvable in ${\mathcal{SAR}}_{\mathcal{J}}^n,$ we derive the generalized representation of the solutions. In addition, in corresponding solution set of the equation, we provide the explicit expression of the nearest matrix to a given matrix in the Frobenius norm.