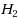Molecular descriptors and quasi-distribution functions

The concept of molecular quantum similarity is a rather subjective notion which depends on the definition of a molecular descriptor. Despite the vast set of options, today the main descriptor in use is the electron density usually provided by the time‐independent Schrödinger equation. In this paper, an alternative definition of quantum similarity in the context of the Wigner formulation of quantum mechanics is suggested, based on a molecular descriptor defined in terms of quasi‐distribution functions. We show that this definition has several important advantages over density based similarities. In particular, it offers a higher detailed description of a quantum system, thus refining the concept of similarity. To support our claim, we first present a theoretical example where two systems which appear to be similar, when using the density as a descriptor, become non‐similar when their quasi‐distributions are involved in the computation of the similarity. Moreover we simulate two quantum systems (utilizing the Wigner Monte Carlo method based on signed particles), i.e. a free Gaussian wave‐packet and an H $H_2$ molecule, and show that Wigner quasi‐distribution functions provide a different perspective on the concept of quantum similarity.