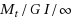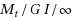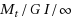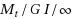| Article ID: | iaor20163717 |
| Volume: | 84 |
| Issue: | 1 |
| Start Page Number: | 111 |
| End Page Number: | 143 |
| Publication Date: | Oct 2016 |
| Journal: | Queueing Systems |
| Authors: | Whitt Ward |
| Keywords: | stochastic processes, markov processes, simulation |
To better understand what stochastic model might be appropriate in applications with system data, we study the consequences of fitting a stationary birth‐and‐death (BD) process to the sample path of a periodic