Recent advances in drift analysis have given us better and better tools for understanding random processes, including the run time of randomized search heuristics. In the setting of multiplicative drift we do not only have excellent bounds on the expected run time, but also more general results showing the strong concentration of the run time. In this paper we investigate the setting of additive drift under the assumption of strong concentration of the ‘step size’ of the process. Under sufficiently strong drift towards the goal we show a strong concentration of the hitting time. In contrast to this, we show that in the presence of small drift a Gambler’s‐Ruin‐like behavior of the process overrides the influence of the drift, leading to a maximal movement of about
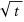steps within t iterations. Finally, in the presence of sufficiently strong negative drift the hitting time is superpolynomial with high probability; this corresponds to the well‐known Negative Drift Theorem.
