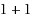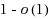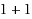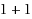| Article ID: | iaor20162555 |
| Volume: | 75 |
| Issue: | 3 |
| Start Page Number: | 507 |
| End Page Number: | 528 |
| Publication Date: | Jul 2016 |
| Journal: | Algorithmica |
| Authors: | Sutton Andrew |
| Keywords: | programming: linear, combinatorial optimization, graphs, heuristics |
The (