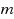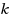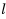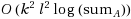| Article ID: | iaor20162393 |
| Volume: | 19 |
| Issue: | 3 |
| Start Page Number: | 285 |
| End Page Number: | 293 |
| Publication Date: | Jun 2016 |
| Journal: | Journal of Scheduling |
| Authors: | Dostert M, Politz A, Schmitz H |
| Keywords: | scheduling, combinatorial optimization |
We show that the following fundamental question in student sectioning can be efficiently decided in polynomial time: Is it possible to assign