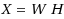Heuristics for exact nonnegative matrix factorization

The exact nonnegative matrix factorization (exact NMF) problem is the following: given an m‐by‐n nonnegative matrix X and a factorization rank r, find, if possible, an m‐by‐r nonnegative matrix W and an r‐by‐n nonnegative matrix H such that 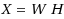$X=WH$ . In this paper, we propose two heuristics for exact NMF, one inspired from simulated annealing and the other from the greedy randomized adaptive search procedure. We show empirically that these two heuristics are able to compute exact nonnegative factorizations for several classes of nonnegative matrices (namely, linear Euclidean distance matrices, slack matrices, unique‐disjointness matrices, and randomly generated matrices) and as such demonstrate their superiority over standard multi‐start strategies. We also consider a hybridization between these two heuristics that allows us to combine the advantages of both methods. Finally, we discuss the use of these heuristics to gain insight on the behavior of the nonnegative rank, i.e., the minimum factorization rank such that an exact NMF exists. In particular, we disprove a conjecture on the nonnegative rank of a Kronecker product, propose a new upper bound on the extension complexity of generic n‐gons and conjecture the exact value of (i) the extension complexity of regular n‐gons and (ii) the nonnegative rank of a submatrix of the slack matrix of the correlation polytope.