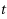Stability and Hopf bifurcation of a HIV infection model with CTL-response delay

In this paper, we consider a HIV infection model with CTL‐response delay and analyze the effect of time delay on stability of equilibria. We obtain the global stability of the infection‐free equilibrium and give sufficient conditions for the local stability of the CTL‐absent equilibrium and CTL‐present equilibrium. By choosing the CTL‐response delay $t$ as a bifurcation parameter, we prove that the CTL‐present equilibrium is locally asymptotically stable in a range of delays and a Hopf bifurcation occurs as $t$ crosses a critical value. Numerical simulations are given to support the theoretical results.