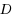| Article ID: | iaor201530668 |
| Volume: | 297 |
| Start Page Number: | 117 |
| End Page Number: | 131 |
| Publication Date: | May 2016 |
| Journal: | Journal of Computational and Applied Mathematics |
| Authors: | Wischerhoff Marius, Plonka Gerlind |
| Keywords: | statistics: sampling |
In this paper, we want to reconstruct polygonal shapes in the real plane from as few Fourier samples as possible, that is, we want to recover an original polygonal domain