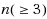This paper deals with dynamic behaviors of Hopfield type neural network model of
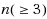identical neurons with two time‐delayed connections coupled in a star configuration. Delay dependent as well as independent local stability conditions about trivial equilibrium is found. Considering synaptic weight and time delay as parameters Hopf‐bifurcation, steady‐state bifurcation and equivariant steady state bifurcation criteria are given. The criterion for the global stability of the system is presented by constructing a suitable Lyapunov functional. Also conditions for absolute synchronization about the trivial equilibrium are also shown. Using normal form method and the center manifold theory the direction of the Hopf‐bifurcation, stability and the properties of Hopf‐bifurcating periodic solutions are determined. Numerical simulations are presented to verify the analytical results. The effect of synaptic weight and delay on different types of oscillations, e.g. in‐phase, phase‐locking, standing wave and oscillation death, has been shown numerically.
