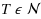Consider a search problem in which a stationary object is in one of
locations. Each location can be searched using one of
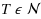technologies, and each location‐technology pair has a known associated cost and overlook probability. These quantities may depend on the number of times that the technology is applied to the location. This paper finds a search policy that maximizes the probability of finding the object given a constraint on the available budget. It also finds the policy that maximizes the probability of correctly stating at the end of a search where the object is. Additionally it exhibits another policy that minimizes the expected cost required to find the object and the optimal policy for stopping.
