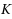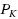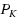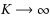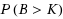| Article ID: | iaor201526336 |
| Volume: | 81 |
| Issue: | 3 |
| Start Page Number: | 299 |
| End Page Number: | 315 |
| Publication Date: | Jun 2015 |
| Journal: | Mathematical Methods of Operations Research |
| Authors: | Zwart Bert |
| Keywords: | simulation, markov processes |
Consider the single‐server queue in which customers are rejected if their total sojourn time would exceed a certain level