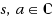| Article ID: | iaor201526149 |
| Volume: | 69 |
| Issue: | 2 |
| Start Page Number: | 253 |
| End Page Number: | 270 |
| Publication Date: | Jun 2015 |
| Journal: | Numerical Algorithms |
| Authors: | Johansson Fredrik |
We study the use of the Euler‐Maclaurin formula to numerically evaluate the Hurwitz zeta function