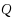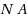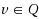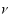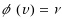| Article ID: | iaor201526326 |
| Volume: | 44 |
| Issue: | 2 |
| Start Page Number: | 377 |
| End Page Number: | 386 |
| Publication Date: | May 2015 |
| Journal: | International Journal of Game Theory |
| Authors: | Edhan Omer |
| Keywords: | graphs |
We prove that every continuous value on a space of vector measure market games