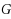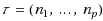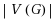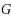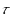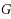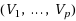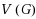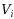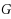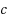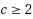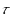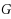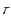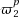On the complexity of partitioning a graph into a few connected subgraphs

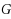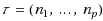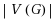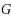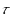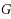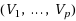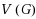Given a graph $G$ , a sequence $\mathit{\tau }=(n_1,\dots ,n_p)$ of positive integers summing up to $|V(G)|$ is said to be realizable in $G$ if there exists a realization of $\mathit{\tau }$ in $G$ , i.e. a partition $(V_1,\dots ,V_p)$ of $V(G)$ such that each 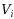$V_i$ induces a connected subgraph of 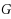$G$ on $n_i$ vertices. 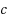We first give a reduction showing that the problem of deciding whether a sequence with $c$ elements is realizable in a graph is NP‐complete for every fixed 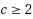$c\ge 2$ . Thanks to slight modifications of this reduction, we then prove additional hardness results on decision problems derived from the previous one. In particular, we show that the previous problem remains NP‐complete when a constant number of vertex‐membership constraints must be satisfied. We then prove the tightness of an easiness result proved independently by Györi and Lovász regarding a similar problem. 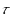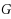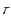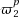We finally show that another graph partition problem, asking whether several partial realizations of $\mathit{\tau }$ in $G$ can be extended to obtain whole realizations of $\mathit{\tau }$ in $G$ , is ${\varpi }_2^p$ ‐complete.