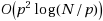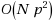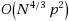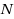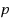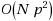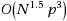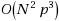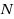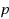Computational cost estimates for parallel shared memory isogeometric multi-frontal solvers

In this paper we present computational cost estimates for parallel shared memory isogeometric multi‐frontal solvers. The estimates show that the ideal isogeometric shared memory parallel direct solver scales as 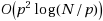$O(p^{2}log(N/p))$ for one dimensional problems, 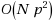$O\left(N{p}^2\right)$ for two dimensional problems, and 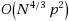$O\left(N^{4/3}{p}^2\right)$ for three dimensional problems, where 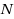$N$ is the number of degrees of freedom, and 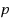$p$ is the polynomial order of approximation. The computational costs of the shared memory parallel isogeometric direct solver are compared with those corresponding to the sequential isogeometric direct solver, being the latest equal to 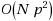$O\left(N{p}^2\right)$ for the one dimensional case, 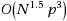$O\left(N^{1.5}{p}^3\right)$ for the two dimensional case, and 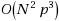$O\left(N^2{p}^3\right)$ for the three dimensional case. The shared memory version significantly reduces both the scalability in terms of 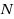$N$ and $p$ . 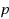Theoretical estimates are compared with numerical experiments performed with linear, quadratic, cubic, quartic, and quintic B‐splines, in one and two spatial dimensions.