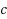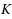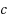| Article ID: | iaor2014917 |
| Volume: | 79 |
| Issue: | 3 |
| Start Page Number: | 273 |
| End Page Number: | 291 |
| Publication Date: | Jun 2014 |
| Journal: | Mathematical Methods of Operations Research |
| Authors: | Avrachenkov Konstantin, Morozov Evsey |
| Keywords: | queues: theory, communications, networks |
We consider a finite buffer capacity GI/GI/c/K‐type retrial queueing system with constant retrial rate. The system consists of a primary queue and an orbit queue. The primary queue has