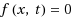Discrete‐time ZD, GD and NI for solving nonlinear time‐varying equations

A special class of neural dynamics called Zhang dynamics (ZD), which is different from gradient dynamics (GD), has recently been proposed, generalized, and investigated for solving time‐varying problems by following Zhang et al.’s design method. In view of potential digital hardware implemetation, discrete‐time ZD (DTZD) models are proposed and investigated in this paper for solving nonlinear time‐varying equations in the form of $f(x,t)=0$ . For comparative purposes, the discrete‐time GD (DTGD) model and Newton iteration (NI) are also presented for solving such nonlinear time‐varying equations. Numerical examples and results demonstrate the efficacy and superiority of the proposed DTZD models for solving nonlinear time‐varying equations, as compared with the DTGD model and NI.