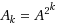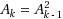Large‐scale Stein and Lyapunov equations, Smith method, and applications

We consider the solution of large‐scale Lyapunov and Stein equations. For Stein equations, the well‐known Smith method will be adapted, with 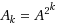$A_k=A^{2^k}$ not explicitly computed but in the recursive form 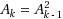$A_k=A_{k‐1}^2$ , and the fast growing but diminishing components in the approximate solutions truncated. Lyapunov equations will be first treated with the Cayley transform before the Smith method is applied. For algebraic equations with numerically low‐ranked solutions of dimension n, the resulting algorithms are of an efficient O(n) computational complexity and memory requirement per iteration and converge essentially quadratically. An application in the estimation of a lower bound of the condition number for continuous‐time algebraic Riccati equations is presented, as well as some numerical results.