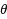Fitted finite volume method for a generalized Black–Scholes equation transformed on finite interval

A generalized Black–Scholes equation is considered on the semi‐axis. It is transformed on the interval (0, 1) in order to make the computational domain finite. The new parabolic operator degenerates at the both ends of the interval and we are forced to use the Gärding inequality rather than the classical coercivity. A fitted finite volume element space approximation is constructed. It is proved that the time $\theta$ ‐weighted full discretization is uniquely solvable and positivity‐preserving. Numerical experiments, performed to illustrate the usefulness of the method, are presented.