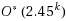| Article ID: | iaor2014187 |
| Volume: | 27 |
| Issue: | 1 |
| Start Page Number: | 3 |
| End Page Number: | 13 |
| Publication Date: | Jan 2014 |
| Journal: | Journal of Combinatorial Optimization |
| Authors: | Zhu Binhai, Jiang Haitao, Zhang Chihao |
| Keywords: | biology |
In this paper, we study the Radiation hybrid map construction (