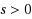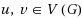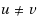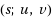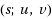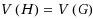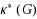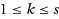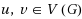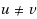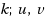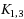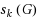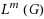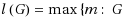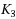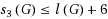For an integer
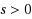 and for
and for
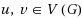 with
with
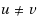 , an
, an
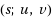 ‐path‐system of G is a subgraph H of G consisting of s internally disjoint (u, v)‐paths, and such an H is called a spanning
‐path‐system of G is a subgraph H of G consisting of s internally disjoint (u, v)‐paths, and such an H is called a spanning
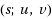 ‐path system if
‐path system if
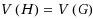 . The spanning connectivity
. The spanning connectivity
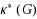 of graph G is the largest integer s such that for any integer k with
of graph G is the largest integer s such that for any integer k with
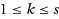 and for any
and for any
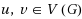 with
with
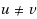 , G has a spanning (
, G has a spanning (
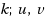 )‐path‐system. Let G be a simple connected graph that is not a path, a cycle or a
)‐path‐system. Let G be a simple connected graph that is not a path, a cycle or a
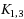 . The spanning k‐connected index of G, written
. The spanning k‐connected index of G, written
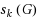 , is the smallest nonnegative integer m such that
, is the smallest nonnegative integer m such that
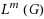 is spanning k‐connected. Let
is spanning k‐connected. Let
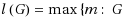 has a divalent path of length m that is not both of length 2 and in a
has a divalent path of length m that is not both of length 2 and in a
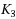 }, where a divalent path in G is a path whose interval vertices have degree two in G. In this paper, we prove that
}, where a divalent path in G is a path whose interval vertices have degree two in G. In this paper, we prove that
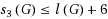 . The key proof to this result is that every connected 3‐triangular graph is 2‐collapsible.
. The key proof to this result is that every connected 3‐triangular graph is 2‐collapsible.