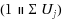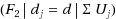Stochastic scheduling with minimizing the number of tardy jobs using chance constrained programming

In this research, two scheduling problems i.e., single machine scheduling problem with minimizing the number of tardy jobs 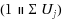$(1\parallel \Sigma U_j)$ and two machine flow shop scheduling problem with a common due date and minimizing the number of tardy jobs 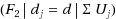$(F_2|d_j=d|\Sigma U_j)$ are investigated in a stochastic setting in the class of non‐preemptive static list policies. It is assumed that the processing times of jobs are independent random variables. The stochastic problems are solved based on chance constrained programming. An equivalent deterministic problem is generated for each stochastic problem by linearization of the chance constraints. Then, the generated deterministic problems are solved using efficient algorithms, which have been developed for the deterministic version of the problems. Several numerical examples are presented to illustrate the solution methods.