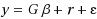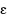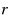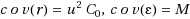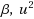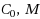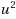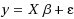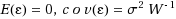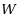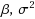The linear model
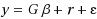 is considered, in which
is considered, in which
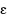 represents measurement noise, and
represents measurement noise, and
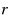 represents ‘process’ noise. The two noise terms are assumed to be independent of one another, zero mean, with
represents ‘process’ noise. The two noise terms are assumed to be independent of one another, zero mean, with
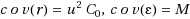 . Here, it is assumed that
. Here, it is assumed that
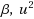 are unknown, but that the matrices
are unknown, but that the matrices
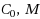 are known. Interest here focuses on inferences for the parameter
are known. Interest here focuses on inferences for the parameter
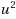 . This may be viewed as a slight generalization of the weighted least squares model
. This may be viewed as a slight generalization of the weighted least squares model
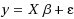 with
with
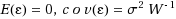 where
where
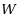 is a known positive definite weighting matrix, and
is a known positive definite weighting matrix, and
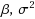 are unknown. This model finds applications in estimating delays through the ionosphere experienced by signals sent by navigation satellites.
are unknown. This model finds applications in estimating delays through the ionosphere experienced by signals sent by navigation satellites.