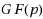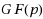| Article ID: | iaor201111214 |
| Volume: | 55 |
| Issue: | 1-2 |
| Start Page Number: | 142 |
| End Page Number: | 150 |
| Publication Date: | Jan 2012 |
| Journal: | Mathematical and Computer Modelling |
| Authors: | Fu Shaojing, Li Chao, Qu Longjiang, Dong Deshuai |
| Keywords: | security |
Rotation symmetric functions which are invariant under circular translation of indices are widely used as components of different cryptosystems. In this paper, we do some enumeration on rotation symmetric functions over