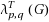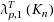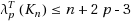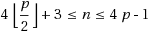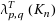Given a graph G and positive integers p,q with p≥q, the (p,q)‐total number
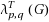 of G is the width of the smallest range of integers that suffices to label the vertices and the edges of G such that the labels of any two adjacent vertices are at least q apart, the labels of any two adjacent edges are at least q apart, and the difference between the labels of a vertex and its incident edges is at least p. Havet and Yu (2008) first introduced this problem and determined the exact value of
of G is the width of the smallest range of integers that suffices to label the vertices and the edges of G such that the labels of any two adjacent vertices are at least q apart, the labels of any two adjacent edges are at least q apart, and the difference between the labels of a vertex and its incident edges is at least p. Havet and Yu (2008) first introduced this problem and determined the exact value of
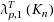 except for even n with p+5≤n≤6p
2−10p+4. Their proof for showing that
except for even n with p+5≤n≤6p
2−10p+4. Their proof for showing that
 for odd n has some mistakes. In this paper, we prove that if n is odd, then
for odd n has some mistakes. In this paper, we prove that if n is odd, then
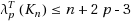 if p=2, p=3, or
if p=2, p=3, or
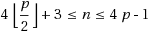 . And we extend some results that were given in Havet and Yu (Discrete Math 308:496–513, 2008). Beside these, we give a lower bound for
. And we extend some results that were given in Havet and Yu (Discrete Math 308:496–513, 2008). Beside these, we give a lower bound for
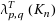 under the condition that q
<p
<2q.
under the condition that q
<p
<2q.