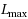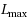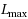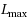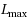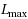| Article ID: | iaor20125438 |
| Volume: | 40 |
| Issue: | 1 |
| Start Page Number: | 328 |
| End Page Number: | 343 |
| Publication Date: | Jan 2013 |
| Journal: | Computers and Operations Research |
| Authors: | Wilson James R, King Russell E, Lobo Benjamin J, Hodgson Thom J, Thoney Kristin A |
| Keywords: | combinatorial optimization, scheduling, networks: flow, heuristics |
A common industrial operation is a dual resource constrained job shop where: (a) the objective is to minimize