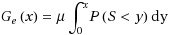Exact simulation of the stationary distribution of the FIFO M/G/c queue: the general case for ρ<c

We present an exact simulation algorithm for the stationary distribution of customer delay for FIFO M/G/c queues in which ρ=λ/μ <c. In Sigman (J. Appl. Probab. 48A:209–216, 2011) an exact simulation algorithm was presented but only under the strong condition that ρ <1 (super stable case). We only assume that the service‐time distribution G(x)=P(S≤x), x≥0, with mean 0<E(S)=1/μ <∞, and its corresponding equilibrium distribution 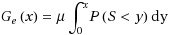$G_e(x)=\mu {\int }_0^{x}P(S<y)\mathrm{dy}$ are such that samples of them can be simulated. Unlike the methods used in Sigman (2011) involving coupling from the past, here we use different methods involving discrete‐time processes and basic regenerative simulation, in which, as regeneration points, we use return visits to state 0 of a corresponding random assignment (RA) model which serves as a sample‐path upper bound.