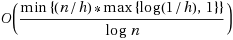Parallel Algorithms for Partitioning Sorted Sets and Related Problems

We consider the following partition problem: Given a set S of n elements that is organized as k sorted subsets of size n/k each and given a parameter h with 1/k ≤ h ≤ n/k , partition S into g = O(n/(hk)) subsets D 1 , D 2 ,… , D g of size θ(hk) each, such that, for any two indices i and j with 1 ≤ i < j ≤ g , no element in D i is bigger than any element in D j . Note that with various combinations of the values of parameters h and k , several fundamental problems, such as merging, sorting, and finding an approximate median, can be formulated as or be reduced to this partition problem. The partition problem also finds many applications in solving problems of parallel computing and computational geometry. In this paper we present efficient parallel algorithms for solving the partition problem and a number of its applications. Our parallel partition algorithm runs in O( log n) time using 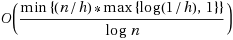$O\left(\frac{\min \left\{\left(n/h\right)*\max \left\{\log \left(1/h\right)\mathrm{,}1\right\}\right\}}{\log n}\right)$ processors in the EREW PRAM model. The complexity bounds of our parallel partition algorithm on the respective special cases match those of the optimal EREW PRAM algorithms for merging, sorting, and finding an approximate median. Using our parallel partition algorithm, we are also able to obtain better complexity bounds (even possibly on a weaker parallel model) than the previously best known parallel algorithms for several important problems, including parallel multiselection, parallel multiranking, and parallel sorting of k sorted subsets.