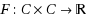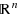Local boundedness of monotone bifunctions

We consider bifunctions 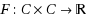$F:C\times C\to ℝ$ where C is an arbitrary subset of a Banach space. We show that under weak assumptions, monotone bifunctions are locally bounded in the interior of their domain. As an immediate corollary, we obtain the corresponding property for monotone operators. Also, we show that in contrast to maximal monotone operators, monotone bifunctions (maximal or not maximal) can also be locally bounded at the boundary of their domain; in fact, this is always the case whenever C is a locally polyhedral subset of 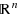${ℝ}^n$ and F(x, ·) is quasiconvex and lower semicontinuous.