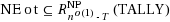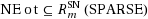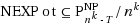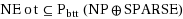| Article ID: | iaor20119249 |
| Volume: | 22 |
| Issue: | 3 |
| Start Page Number: | 482 |
| End Page Number: | 493 |
| Publication Date: | Oct 2011 |
| Journal: | Journal of Combinatorial Optimization |
| Authors: | Fu Bin, Li Angsheng, Zhang Liyu |
| Keywords: | combinatorial optimization |
We investigate the question whether NE can be separated from the reduction closures of tally sets, sparse sets and NP. We show that (1)