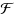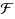The core of a cooperative game on a set of players N is one of the most popular concepts of solution. When cooperation is restricted (feasible coalitions form a subcollection
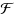of 2
N
), the core may become unbounded, which makes its usage questionable in practice. Our proposal is to make the core bounded by turning some of the inequalities defining the core into equalities (additional efficiency constraints). We address the following mathematical problem: can we find a minimal set of inequalities in the core such that, if turned into equalities, the core becomes bounded? The new core obtained is called the restricted core. We completely solve the question when
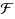is a distributive lattice, introducing also the notion of restricted Weber set. We show that the case of regular set systems amounts more or less to the case of distributive lattices. We also study the case of weakly union‐closed systems and give some results for the general case.
