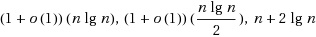Reconstruction of hidden graphs and threshold group testing

Classical group testing is a search paradigm where the goal is the identification of individual positive elements in a large collection of elements by asking queries of the form ‘Does a set of elements contain a positive one?’. A graph reconstruction problem that generalizes the classical group testing problem is to reconstruct a hidden graph from a given family of graphs by asking queries of the form ‘Whether a set of vertices induces an edge’. Reconstruction problems on families of Hamiltonian cycles, matchings, stars and cliques on n vertices have been studied where algorithms of using at most 2nlgn,(1+o(1))(nlgn),2n and 2n queries were proposed, respectively. In this paper we improve them to $(1+o(1))(n\lg n),(1+o(1))(\frac{n\lg n}{2}),n+2\lg n$ and n+lgn, respectively. Threshold group testing is another generalization of group testing which is to identify the individual positive elements in a collection of elements under a more general setting, in which there are two fixed thresholds ℓ and u, with ℓ<u, and the response to a query is positive if the tested subset of elements contains at least u positive elements, negative if it contains at most ℓ positive elements, and it is arbitrarily given otherwise. For the threshold group testing problem with ℓ=u-1, we show that p positive elements among n given elements can be determined by using O(plgn) queries, with a matching lower bound.