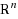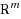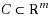| Article ID: | iaor20117328 |
| Volume: | 214 |
| Issue: | 3 |
| Start Page Number: | 485 |
| End Page Number: | 492 |
| Publication Date: | Nov 2011 |
| Journal: | European Journal of Operational Research |
| Authors: | Villacorta Kely D V, Oliveira P Roberto |
| Keywords: | vector optimization |
This paper studies the vector optimization problem of finding weakly efficient points for maps from