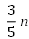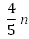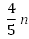| Article ID: | iaor20103514 |
| Volume: | 57 |
| Issue: | 3 |
| Start Page Number: | 499 |
| End Page Number: | 516 |
| Publication Date: | Jul 2010 |
| Journal: | Algorithmica |
| Authors: | Uno Takeaki, Okamoto Yoshio, Christ Tobias, Hoffmann Michael |
| Keywords: | art gallery problem |
We consider a novel class of art gallery problems inspired by wireless localization that has recently been introduced by Eppstein, Goodrich, and Sitchinava. Given a simple polygon