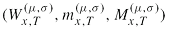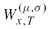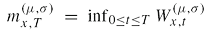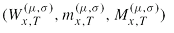Exact simulation of final, minimal and maximal values of Brownian motion and jump-diffusions with applications to option pricing

We introduce a method for generating 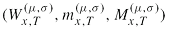${\displaystyle (W_{x,T}^{(\mu ,\sigma )},m_{x,T}^{(\mu ,\sigma )},M_{x,T}^{(\mu ,\sigma )})}$ , where 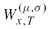${\displaystyle W_{x,T}^{(\mu ,\sigma )}}$ denotes the final value of a Brownian motion starting in x with drift μ and volatility σ at some final time T, 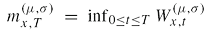${\displaystyle m_{x,T}^{(\mu ,\sigma )}={\inf }_{0\le t\le T}{W}_{x,t}^{(\mu ,\sigma )}}$ and ${\displaystyle M_{x,T}^{(\mu ,\sigma )}={\backslash \sup }_{0\le t\le T}{W}_{x,t}^{(\mu ,\sigma )}}$ . 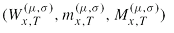By using the trivariate distribution of ${\displaystyle (W_{x,T}^{(\mu ,\sigma )},m_{x,T}^{(\mu ,\sigma )},M_{x,T}^{(\mu ,\sigma )})}$ , we obtain a fast method which is unaffected by the well-known random walk approximation errors. The method is extended to jump-diffusion models. As sample applications we include Monte Carlo pricing methods for European double barrier knock-out calls with continuous reset conditions under both models. The proposed methods feature simple importance sampling techniques for variance reduction.